Maxwell's equations can be used to derive the laws of reflection and refraction, which tell us how light waves behave at the boundary between two media with different indices of refraction.  In 1650, Fermat discovered a way to explain reflection and refraction as the consequence of one single principle.  It is called the principle of least time or Fermat's principle.

Assume we want light to get from point A to point B, subject to some boundary condition.  For example, we want the light to bounce off a mirror or to pass through a piece of glass on its way from A to B.  Fermat's principle states that of all the possible paths the light might take, that satisfy those boundary conditions, light takes the path which requires the least amount of time.

Consider the diagram on the right.  We want light to leave point A, bounce off the mirror, and get to point B.  Let the perpendicular distance from the mirror of both A and B be d and the shortest distance between the points be D.  Assume that light takes the path shown.  The length of this path is

L = (x² + d²)^½ + ((D - x)² + d²)^½.

Since the speed of light is the same everywhere along all possible paths, the shortest path requires the shortest time.  To find the shortest path, we differentiate L with respect to x and set the result equal to zero.  (This yields an extremum in the function L(x).)

dL/dx = x/(x² + d²)^½  -  (D - x)/((D - x)² + d²)^½ = 0.

x²/(x² + d²)  ⁼  (D²  + x² - 2Dx)/(D² + x² - 2Dx + d²).
x²(D² + x² - 2Dx + d²)  ⁼  (D²  + x² - 2Dx)(x² + d²).

After canceling equal terms on both sides we are left with d²D² = 2Dx,   or x = D/2.
The path that takes the shortest time is the one for which x = D/2, or equivalently, the one for which θ_i = θ_r.  Fermat's principle yields the law of reflection.

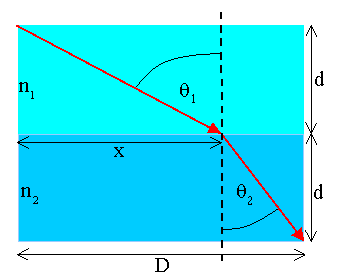Now assume we want light to propagate from point A to point B across the boundary between medium 1 and medium 2.  For the path shown in the figure on the right the time required is

t = (x² + d²)^½/(c/n₁) + ((D - x)² + d²)^½/(c/n₂).

Setting dt/dx = 0 we obtain

n₁ x/(x² + d²)^½ = n₂ (D - x)/((D - x)² + d²)^½

or n₁sinθ₁ = n₂sinθ₂.

Fermat's principle yields Snell's law.

Embedded  Question 1

Consider consider two people near the ocean.

• In situation A person 1 is on the beach and person 2 is in the water.  Person 1 must reach person 2 as quickly as possible.  (A lifeguard may have to get to a swimmer in distress.)  Which path should  person 1 take?  Make qualitative arguments. Assume that person 1 can run faster on the beach than swim in the ocean.
• In situation B person 1 and person 2 are both on the beach.  Person 1 must reach person 2 as quickly as possible while first getting some ocean water.  (Maybe a fire needs to be put out.)  Which path should  person 1 take?  Make qualitative arguments.

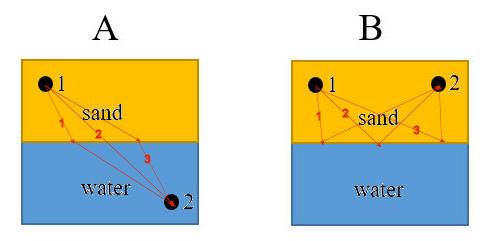
Discuss this with your fellow students in the discussion forum!
Consider the speed of of person 1 on the sand and in the water. 
Which path takes the least amount time in situation A and in situation B.

Light can travel faster in air than in water.  The qualitative arguments you make about the path that takes the least amount of time for the person near the sand-water interface in situations A and B also apply to the path of light light entering from air into water or reflecting from the air-water interface.  That is Fermat's principle.  Fermat's principle leads automatically to the principle of ray reversibility in geometrical optics.  It does not matter if you are going from A to B or B to A, the path that takes the least amount of time is the same.