A neutral, straight, conducting wire contains equal amounts of positive and
negative charges. However, the electrons are free to move inside the wire,
while the positive nuclei are not.A neutral, straight, conducting wire contains equal amounts of positive and
negative charges. However, the electrons are free to move inside the wire,
while the positive nuclei are not.
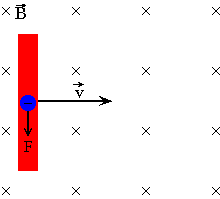
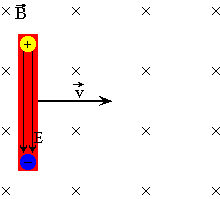
If a straight conducting wire is placed in a plane perpendicular to a uniform
magnetic field, and is moving in a direction perpendicular to the field, then
each charge q in the wire experiences a magnetic force of magnitude F = qvB. The negatively charged electrons
will accelerate in response to this force. Since they cannot leave the
wire, negative charge will accumulate on one end of the wire, while positive
charge will be left behind on the other end. The separated charges produce
an electric field, which exerts a force on the other charges in the wire.
This electric force opposes the magnetic force. Once the electric force is
strong enough to cancel the magnetic force, electrons will no longer accelerate,
and their net motion will stop due to the resistance of the wire. We then
have qvB = qE. The electric field in the wire is equal to E = vB.
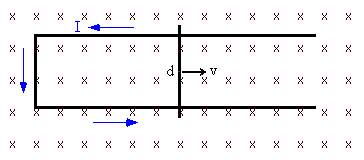If we place the wire on a conducting rail, a current will start to flow in
the circuit formed by the rail and the wire.
The emf driving the current is equal to vB times the length d of the section
of wire connecting the rails. (The work done per unit charge (voltage) is
vBd, when a charge moves from one end of the moving wire to the other end.)
The current flowing in the circuit will be I = vBd/R, where R is the resistance of the circuit.
In the above "filamentary" circuit (consisting only of wires or rods) the
motional emf = B*d*v.
The magnetic flux through the circuit at time t is ΦB = B*A = B*L*d,
where L is the length of the circuit at time t.
The rod moves with speed v.
The rate at which the flux changes is dΦB/dt = B*d*dL/dt =
B*d*v, since the only thing changing is the length of the circuit, and ΔL/∆t = v.
We can therefore write
dΦB/dt (filamentary circuit with moving parts, constant B) = motional emf.
Motional emf is not induced emf. The flux of the magnetic field though a fixed area does not change. Instead, an external force does work moving wires that are part of a circuit in a constant magnetic field. But for filamentary circuits we can write down one mathematical equation that expresses both Faraday's law and motional emf.
dΦB/dt (any flux changes through filamentary circuit) = emf.
In this equation emf stands for motional and induced emf.
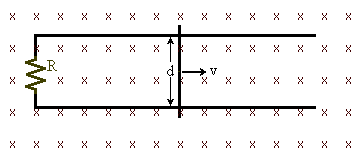Problem:In the figure on the right, assume that R = 6 Ω, d = 1.2 m, and a uniform 2.5 T magnetic field is directed into the page. At what speed should the bar be moved to produce 0.5 A in the resistor?
Solution:
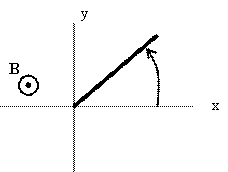
Problem:A conducting rod of length L lying in the xy-plane pivots with constant angular velocity ω counterclockwise about the origin. A constant magnetic field of magnitude B0 is oriented in the z-direction. Will a motional emf be induced in the rod? If yes, which end of the rod will have a positive voltage?
Solution:
In
this
video clip
a thin rod is moved quickly in a magnetic field produced by a set of magnets.
The north pole of the magnets points up. If the current through the rod from
left to right, then it flows from the red lead through the meter to the black
lead, then the deflection of the needle of the meter is to the right.
You can verify the directional aspects of motional emf.