Simple harmonic motion:
A = amplitude
T = period
ω = angular frequency
f = frequency
f = 1/T
ω = 2π/T = ω = 2πf
An object whose position as a function of time
varies as
x(t) = Acos(ωt) exhibits simple harmonic motion.
The
velocity of the object as a function of time is given by
v(t) = -ωAsin(ωt), and the acceleration is given by
a(t) = -ω2Acos(ωt) = -ω2x.
F = -kx = mω2x.
ω2 = k/m,
ω = √(k/m), T = 2π√(m/k),
f = (1/2π)√(k/m).
Simple harmonic motion is accelerated motion.
If an object exhibits simple harmonic motion if it is acted on by a restoring force F = -kx, with k = mω2.
A mass oscillating on a spring is an example of an object moving with
simple harmonic motion.
The pendulum:
 Most system which
have an equilibrium position execute simple harmonic motion about this
position when they are displaced from equilibrium, as long as the
displacements are small. The restoring forces approximately obey
Hooke's law. However, for larger displacements the systems become
anharmonic oscillators, i.e. the
restoring forces are no longer proportional to the displacements. The
period then depends on the amplitude. A familiar example of such a
system is the simple pendulum.
Most system which
have an equilibrium position execute simple harmonic motion about this
position when they are displaced from equilibrium, as long as the
displacements are small. The restoring forces approximately obey
Hooke's law. However, for larger displacements the systems become
anharmonic oscillators, i.e. the
restoring forces are no longer proportional to the displacements. The
period then depends on the amplitude. A familiar example of such a
system is the simple pendulum.
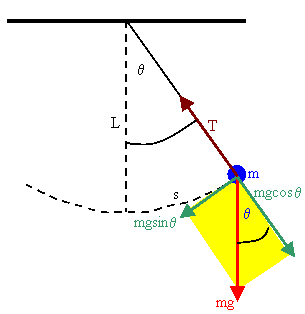
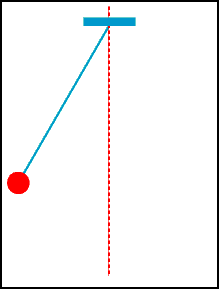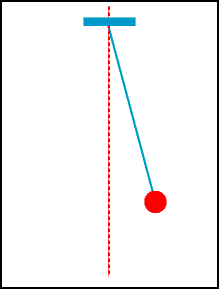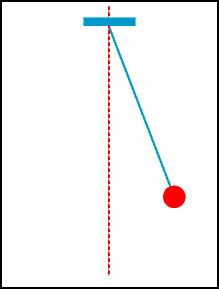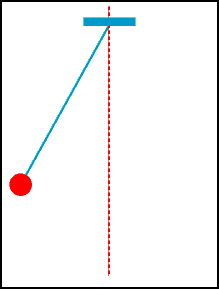 An ideal simple pendulum consists of a point mass m suspended from a
support by a massless string of length L. (A good approximation is a
small mass, for example a sphere with a diameter much smaller than L,
suspended from a light string.) The equilibrium position of the mass is
a distance L below the support. The net force acting on m is F=
-mgsinθ. It is a restoring force. The displacement from
the equilibrium position is s = Lθ.
An ideal simple pendulum consists of a point mass m suspended from a
support by a massless string of length L. (A good approximation is a
small mass, for example a sphere with a diameter much smaller than L,
suspended from a light string.) The equilibrium position of the mass is
a distance L below the support. The net force acting on m is F=
-mgsinθ. It is a restoring force. The displacement from
the equilibrium position is s = Lθ.
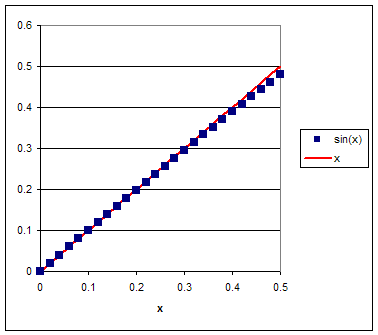 When the displacement from equilibrium is
small, then sinθ ~ θ in radians. (See graph.)
When the displacement from equilibrium is
small, then sinθ ~ θ in radians. (See graph.)
Then F = -mgθ = -(mg/L)s.
The force obeys Hooke's law, F = -ks, with k = mg/L.
For small displacements s the pendulum executes simple harmonic motion
s(t) = smaxcos(ωt
+ φ), with ω2 = g/L.
For small oscillations the period of
a simple pendulum therefore is given by T = 2π/ω = 2π√(L/g).
It is independent of the mass m of the bob. It depends only on the
strength of the gravitational acceleration g and the length of the string L.
By measuring the length and the period of a simple pendulum we can determine
g.
Problem:
Simple pendulum:
F = -mgsinθ,
s = Lθ.
For small displacements:
F = -mgθ = -(mg/L)s,
k = (mg/L),
ω2 = k/m = g/L,
T = 2π√(L/g), f = 1/T.
The angular displacement of a pendulum is represented by the equation
θ = 0.32*cos(ωt) where θ is in radians and ω = 4.43 rad/s.
Determine the period and length of the pendulum.
Solution:
- Reasoning:
θ(t) = θmaxcos(ωt + φ) for small
oscillations.
- Details of the calculation:
Here ω = 4.43/s, ω2 = g/L = 19.62/s2, L = 0.5 m.
T = 2π/ω = 1.42 s
Problem:
A simple pendulum on a cuckoo clock is 5.00 cm long. What is
its frequency?
Solution:
- Reasoning:
For a simple pendulum ω2 = g/L.
- Details of the calculation:
f = 1/T = ω/(2π) = (g/L)1/2/(2π) = 2.23/s.
Problem:
A simple pendulum with a period of 2.00000 s in one location where g
= 9.80 m/s2 is moved to a new location where the period is
now 1.99796 s. What is the acceleration due to gravity at its new
location?
Solution:
- Reasoning:
For a simple pendulum the period T = 2π)/ω is proportional to 1/g1/2.
- Details of the calculation:
Told/Tnew = (gnew/gold)1/2.
gnew = gold*(Told/Tnew)
= 9.8*(2/1.99796)2 = 9.82 m/s2.
Problem:
Pendulum clocks are made to run at the correct rate by adjusting the
pendulum's length. Suppose you move from one city to another where
the acceleration due to gravity is slightly greater, taking your
pendulum clock with you, will you have to lengthen or shorten the
pendulum to keep the correct time, other factors remaining constant?
Solution:
- Reasoning:
For a simple pendulum the period T is proportional to (L/g)1/2.
If g increases slightly, L has to increase slightly to keep the period the
same. You will have to lengthen the pendulum.
External link: A
simple pendulum
 Most system which
have an equilibrium position execute simple harmonic motion about this
position when they are displaced from equilibrium, as long as the
displacements are small. The restoring forces approximately obey
Hooke's law. However, for larger displacements the systems become
anharmonic oscillators, i.e. the
restoring forces are no longer proportional to the displacements. The
period then depends on the amplitude. A familiar example of such a
system is the simple pendulum.
Most system which
have an equilibrium position execute simple harmonic motion about this
position when they are displaced from equilibrium, as long as the
displacements are small. The restoring forces approximately obey
Hooke's law. However, for larger displacements the systems become
anharmonic oscillators, i.e. the
restoring forces are no longer proportional to the displacements. The
period then depends on the amplitude. A familiar example of such a
system is the simple pendulum. An ideal simple pendulum consists of a point mass m suspended from a
support by a massless string of length L. (A good approximation is a
small mass, for example a sphere with a diameter much smaller than L,
suspended from a light string.) The equilibrium position of the mass is
a distance L below the support. The net force acting on m is F=
-mgsinθ. It is a restoring force. The displacement from
the equilibrium position is s = Lθ.
An ideal simple pendulum consists of a point mass m suspended from a
support by a massless string of length L. (A good approximation is a
small mass, for example a sphere with a diameter much smaller than L,
suspended from a light string.) The equilibrium position of the mass is
a distance L below the support. The net force acting on m is F=
-mgsinθ. It is a restoring force. The displacement from
the equilibrium position is s = Lθ. When the displacement from equilibrium is
small, then sinθ ~ θ in radians. (See graph.)
When the displacement from equilibrium is
small, then sinθ ~ θ in radians. (See graph.)