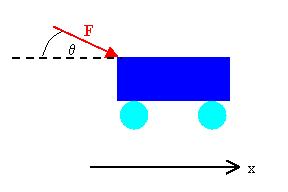
 A shopper in a supermarket pushes a cart with a force of 35 N
directed at an angle of 25o downward from the horizontal.
Find the work done by the shopper as he moves down an aisle of 50 m
length.
A shopper in a supermarket pushes a cart with a force of 35 N
directed at an angle of 25o downward from the horizontal.
Find the work done by the shopper as he moves down an aisle of 50 m
length. Assume you are lifting an object with mass 20 kg from the ground to a height
of 1.5 m. Assume that you are exerting a constant force in the upward
direction and that you are moving the object upward with uniform velocity. The
net force on the object is zero. The force you are exerting is equal in
magnitude and opposite in direction to the force of gravity. As you are lifting
the object you are doing work on the object.
The work W done on an object by a constant force is defined as W =
F·d. It is equal to the magnitude of the force, multiplied by the distance the object
moves in the direction of the force.
In the example above F = mg = (20 kg)(9.8 m/s2) = 196 N, W = (196
N)(1.5 m) = 294 Nm.
Work is a scalar, a number with units. The SI unit of work Nm =
Joule (J).
Work is the "scalar product" or "dot product" of the force and the displacement vector. The scalar product of two vectors A and B is a scalar quantity (a number with units) equal to the product of the magnitudes of the two vectors and the cosine of the smallest angle between them.
A·B = ABcosθ.
In terms of the Cartesian components of the vectors A and B the scalar product is written as
A·B = AxBx + AyBy + AzBz.
In one dimension, the scalar product is positive if the two vectors are
parallel to each other, and it is negative if the two vectors are anti-parallel
to each other, i.e. if they point in opposite directions.
The work done by a force can be positive or negative. If the component
of the force in the direction of the displacement is positive, the work is
positive, and if the component of the force in the direction of the displacement
is negative, the work is negative.
Assume you forgot to set the parking break, and your car starts rolling down a hill. You try in vain to stop it by pulling as hard as you can on the bumper, but the car keeps on moving forward. You exert a force on the car opposite to the direction of travel. The distance traveled in the direction of the force is negative, you do negative work on the car. But the car is pulling you in the direction of travel with a force of equal magnitude (Newton's third law). The car is doing positive work on you.
While mowing a lawn, a boy pushes a lawn mower a total distance of 350 m over the grass with a force of 90 N directed along the horizontal. How much work is done by the boy?
Solution:
 A shopper in a supermarket pushes a cart with a force of 35 N
directed at an angle of 25o downward from the horizontal.
Find the work done by the shopper as he moves down an aisle of 50 m
length.
A shopper in a supermarket pushes a cart with a force of 35 N
directed at an angle of 25o downward from the horizontal.
Find the work done by the shopper as he moves down an aisle of 50 m
length.
Solution:
 Ramps
RampsAssume you want to lift your motorcycle onto the bed of
your pickup truck. You probably will use a ramp. You will choose a
long board. One end of the board will rest on the bed of the truck and
the other end will rest on the ground. You will roll your motorcycle up
this ramp.
You apply a force Fa equal in magnitude and
opposite in direction to the component of the gravitational force
parallel to the ramp. The magnitude of Fa is mgsinθ. You
move the motorcycle a distance h/sinθ in the direction of the force.
The work you do is W = F·d = mgh, where mg is the weight of your
motorcycle and h is the height of the bed. If you would lift the
motorcycle straight up, you would exert a force equal to its weight
through a distance h. When rolling it up the ramp, you have to push on
it through a much longer distance. To do the same work, you therefore
need a much smaller force. The ramp provides mechanical advantage.
Work = large force × small distance = small force × large distance.
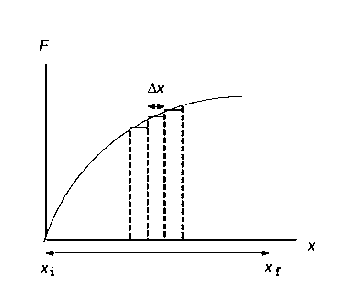 The work done by a varying force is defined as W = Σxixf
Fx∆x, as ∆x becomes
infinitesimally small. (The symbol Σ stand for the sum. Σxixf
Fx∆x is the sum of the products Fx∆x from the
initial to the final position in steps of ∆x.) We can plot the component of the force Fx
acting on the object at position x versus the position x.
The work done by a varying force is defined as W = Σxixf
Fx∆x, as ∆x becomes
infinitesimally small. (The symbol Σ stand for the sum. Σxixf
Fx∆x is the sum of the products Fx∆x from the
initial to the final position in steps of ∆x.) We can plot the component of the force Fx
acting on the object at position x versus the position x.
The work done by the force is equal to the area under the curve.
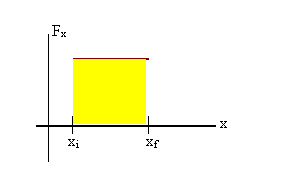 A plot for a constant force acting from xi
to xf is shown on the right.
A plot for a constant force acting from xi
to xf is shown on the right.
The work done by the force
is W = Fx(xf - xi).
In order to lift an object of mass m so that its height increases by a
distance h, you have to exert an average force mg through a distance h.
The work you have to do lifting the object is W = mgh.
A 100 N crate sits on the ground and is attached to one end of a rope.
A person on a balcony pulls up on the rope with a constant force of 100 N
lifting the crate a distance of 3 m.
(a) How much work is done by the person?
(b) How much work is done by gravity?
Solution:
(a) Calculate the work done on a 1500 kg elevator car by
its cable to lift it 40 m at constant speed, assuming friction force averages
100 N.
(b) What is the work done on the elevator car by
the gravitational force in this process?
Solution:
 Work involved in stretching or compressing a spring
Work involved in stretching or compressing a springIn order to compress or stretch a spring, you have to do work. You must
exert a force on the spring equal in magnitude to the force the spring exerts on
you, but opposite in direction. The force you exert is in the direction
of the displacement x from its equilibrium position, Fext = kx. You therefore do work.
Work = area under the curve = width * average height
= (x2 - x1)*k(x2
+ x1)/2 = ½k(x22 - x12).
The work you do when stretching or compressing a spring a distance x from its
equilibrium position therefore is W =
½kx2.
When a 4 kg mass is hung vertically on a certain light spring that obeys
Hooke's law, the spring stretches 2.5 cm. If the
4 kg mass is removed,
(a) how far will the spring stretch if a 1.5 kg
mass is hung on it, and
(b) how much work must an external agent do to stretch the same spring 4 cm
from its unstretched position?
Solution:
If it takes 4 J of work to stretch a Hooke's law spring 10 cm from its unstretched length, determine the extra work required to stretch it an additional 10 cm.
Solution: