What is light?

So light is a stream of photons!  But why then does it act like a wave?  Why do we observe diffraction and interference?

imagePhotons definitely do not behave like macroscopic particles, even very small macroscopic particles.

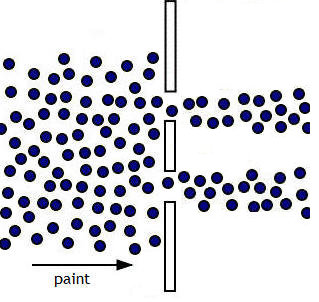
Consider very small macroscopic particles, such as paint droplets from a paint gun.  If we positioned a mask with two slits in front of a wall and used a paint gun to spray paint through the slits onto the wall, the paint does not produce an interference pattern on the wall.  If the slits are sufficiently spaced apart, we observe two sharp lines of paint on the wall, images of the two slits through which the paint was sprayed.  If the spray is very weak and we may see droplet arrive individually, but the droplets will eventually overlap and merge into the two lines.  If the slits are very closely spaced the two lines may merge into just one line.
Even if we make the slits as narrow as possible and put them as close together as possible for the paint droplets to still pass through them without clogging them up, we will only see one line.  We will not observe  an interference pattern.

The figure on the right shows the pattern we expect to observe when paint is sprayed through two slits sufficiently spaced apart.

When we send a beam of photons through a single slit, we observe a single-slit diffraction pattern on a screen.  If we lower the intensity of the beam and use a detector that can detect single photos, we can observe single photons arriving one at a time at seemingly random locations, but over time they will build up a single-slit diffraction pattern.

imageWhen we send a beam of photons through two closely spaced slits, we observe an double-slit interference pattern in the regions where there are no single slit diffraction minima.  If we lower the intensity of the beam and use a detector that can detect single photos, we can observe single photons arriving one at a time at seemingly random locations, but over time they will build up a double-slit interference pattern.

If we device an experiment that allows us to determine which slit the photon went through, the interference pattern vanishes and just the diffraction pattern remains.

 

Simulate the experiment (Excel, download and allow macro to run.)

The linked Excel spreadsheet lets you simulate a double slit experiments using a detector that can detect individual photons.  The two slits are 50 micrometer wide and 150 micrometer apart.  The distance from the slits to the screen is 1 m.  The pattern is a product of a single slit and a double slit diffraction pattern.  Individual photons arrive at the screen.  After many photons have arrived, the interference pattern emerges.  You can vary the wavelength and intensity of the incident light.
(Observe that some interference maxima are missing because they fall onto diffraction minima.)

How is it possible for light to propagate as if it were a wave and yet to be detected as if it were a particle?  How can a single particle interfere with itself?  This paradox is the central theme in Richard Feynman's introduction to the fundamentals of quantum mechanics:

"We choose to examine a phenomenon which is impossible, absolutely impossible, to explain in any classical way, and which has in it the heart of quantum mechanics.  In reality, it contains the only mystery.  We cannot make the mystery go away by explaining how it works . . . In telling you how it works we will have told you about the basic peculiarities of all quantum mechanics."

So what is light, an electromagnetic wave or a stream of photons?  What is our current understanding of the nature of light?

Photons are quanta or packets of energy.  But these quanta behave nothing like macroscopic particles.

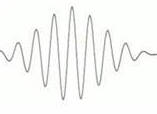imageTo track individual photons or groups of photons, the EM wave we use to predict their behavior must be a wave pulse or wave packet.  A wave packet has finite extend, and the photons will be found in regions where the wave packet has non-zero amplitude.  But only waves that extend forever in space and time have a precisely defined wavelength or frequency.  The wavelength and frequency of wave packets that are supposed to describe a fairly well localized wave pulse cannot be precisely known, but only within some uncertainty.
In Physics 221 you analyzed sound waves.  Using Fourier analysis you found that wave packets of sound contain many different wavelengths or frequencies.

Fourier analysis shows that for all wave packet the product of the uncertainties (spread) in their wave number k = 2π/λ and in their length in space x must be on the order of or larger than 1.

We write  Δx Δk ~ 1.

Similarly Fourier analysis shows that for all wave packets the product of the uncertainties (spread) in their angular frequency ω = 2πf and in their length in time t must be on the order of or larger than 1.

We write  Δt Δω ~ 1.

Since for photons the energy is E = hf and momentum is p = h/λ, we cannot precisely know the energy and momentum of the individual photons in a wave packet.  Rewriting k = 2π/λ = (2π/h)p, we find

Δx Δp ~ h/2π. 

This is the famous Heisenberg uncertainty principle.  Here we are applying it to a photon.  For a photon, we cannot predict its position and momentum with absolute certainty.  The product of the uncertainties is on the order of h/2π or greater.  The quantity h/2π is often denoted by ћ (hbar), ћ = 1.054*10-34 Js.

Rewriting ω = 2πf = (2π/h)E, we find

Δt ΔE ~ h/2π.

This is another form of the uncertainty principle.  If there is a limit to the observation time, there will be an uncertainty in the energy.

Problem:

A pulsed laser produces femtosecond (10-15 s) pulses of near infrared light with 780 nm nominal wavelength.  What is the minimum range of energies of the photons in the pulses.

Solution: