Images can be formed by reflection and by refraction. 

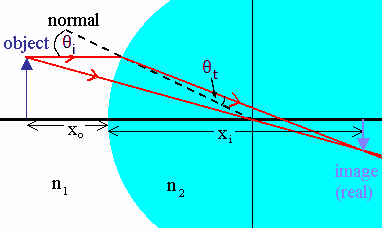
A spherical interface

Assume that we have a boundary between two media with different indices of refraction n₁ and n₂, (n₁ < n₂), and that the boundary has a radius of curvature R, as shown in the figure below.  The center of curvature is at the origin.  Light is refracted at the boundary.  Two rays starting at an object in medium 1 are shown.  One ray is incident on the boundary normally and is not refracted.  The other ray is incident on the boundary at an angle θ_i with respect to the normal, and the refracted angle θ_t is given by Snell's law, n_isinθ_i = n_tsinθ_t.
A real, inverted image is formed where the two rays intersect in medium 2.

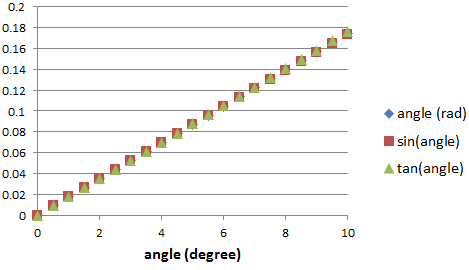For small angles θ, (θ(rad) << 1) we have sinθ ≈ tanθ ≈ θ.
(See the graph on the right which plots θ(rad), sinθ, and tanθ versus θ(deg) for angles from 0 to 10^o.)
This is called the small angle approximation.  It simplifies calculations if it is applicable.

Using the small angle or paraxial approximation we can show that for a spherical interface

n₁/x_o + n₂/x_i = (n₂ - n₁)/R,

if we adopt the following sign conventions.

• The object's position x_o is in front of the interface and the object distance is positive.
• The image distance x_i is positive if the image position lies behind the interface.
• The image distance x_i is negative if the image position lies in front of the interface.
• The radius of curvature R is positive, if the center of curvature lies behind the interface.
• The radius of curvature R is negative, if the center of curvature lies in front of the interface.

The magnification is M = h_i/h_o = -(n₁x_i)/(n₂x_o).  If M is negative, the image is inverted.

If medium 1 is air and medium 2 is a transparent material such a water or glass, can you think of situations where we want to produce a real image inside medium 2 of an object located in medium 1?   See the problem below!

Problem:

A simple model of the human eye ignores its lens entirely.  Much of what the eye does to light happens at the transparent cornea.  Assume that this outer surface has a radius of curvature of 6 mm, and assume that the eyeball contains just one fluid of index of refraction n = 1.4.  Prove that every distant object will be imaged on the retina, 21 mm behind the cornea.  Describe the image.

Solution:

• Reasoning:
  For a single spherical interface we use n₁/x_o + n₂/x_i = (n₂ - n₁)/R.
  For distant objects x_o is very large and n₁/x_o is very small. 
  We can safely ignore this term in the equation n₁/x_o + n₂/x_i = (n₂ - n₁)/R.
  We then have n₂/x_i = (n₂ - n₁)/R.
  R is positive since the center of curvature lies behind the interface.
• Details of the calculation:
  n₂/x_i = (n₂ - n₁)/R  yields x_i = n₂R/(n₂ - n₁) = (1.4*6 mm)/0.4 = 21 mm. 
  The image distance is 21 mm.
  The image is very small and inverted.

Using the principle of ray reversibility, we can switch out object and image.  Let us look at a fish in a water filled spherical bowl.   Water has an index of refraction n₂ = 1.33, so we cannot produce a real image of the fish in front of the bowl.  When looking at the fish from the outside, we will see a upright, enlarged virtual image.

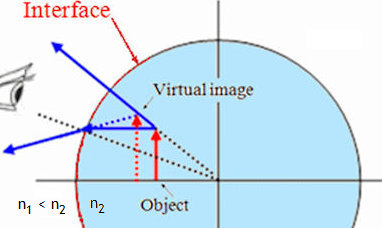
The images below show a frog in a spherical bowl before and after water has been poured into the bowl.  The camera is stationary.  When the frog is under water, the camera takes a picture of an an upright, enlarged, virtual image of the frog.

	Watch as the water is poured into the bowl.
Frog on empty bowl		Frog under water in bowl

A flat interface

For a flat interface we have R = infinite and n₁/x_o + n₂/x_i = 0.
Let an object be underwater, 10 cm from the water-air interface.
We find x_i = -(n₂/n₁)x_o = -(1/1.33)x_o.  x_i = -7.5 cm.
The magnification is M = 1, it is positive.
The image is a virtual, non-inverted image.
A detector viewing the object from the air side sees the image at the apparent position closer to the interface.

The images below were taken without moving the camera.  A coin is placed in each of two empty bowl.  Then water is poured first into the right and then into the left bowl.  When the coin is under water, the camera takes a picture of the virtual image of the coin. The coin appears closer to the camera when underwater.  (You can verify this yourself.)

Problem:

A cubical block of ice, 50 cm on a side, is placed on a level floor over a speck of dust.  Find the location of the image of the dust if the index of refraction of the ice is n = 1.309.

Solution:

• Reasoning:
  For a flat interface we have n₁/x_o + n₂/x_i = 0.
• Details of the calculation:
  x_o = 50 cm.  x_i = -(1/1.309)x_o = -38.2 cm.
  The apparent position is 38.2 cm below the top surface of the ice.