Refraction and reflection

In this lab you will explore the behavior of light at the boundary between two transparent media with different indices of refraction.  A fraction of the incident intensity will be reflected, and the rest of the light will be transmitted.  The direction of propagation of the reflected and transmitted light is given by the laws of reflection and refraction.

• Law of reflection:  θ_i = θ_r
• Snell's law or law of refraction:  n_isinθ_i = n_tsinθ_t.

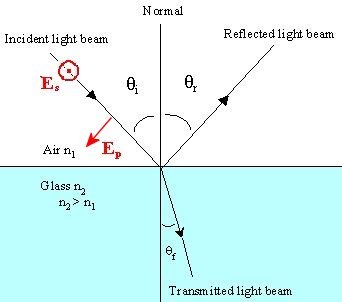How much of the light is reflected and how much is transmitted?

The reflectance R is the ratio of the reflected flux to the incident flux, and the transmittance T is the ratio of the transmitted flux to the incident flux.  Energy conservation requires that R + T = 1 (if there is no absorption).
R and T depend on the indices of refraction of the two media n₁ and n₂, the angle of incidence θ_i, and the polarization of the incident light.
We distinguish between p-polarization and s-polarization.

Consider, for example, an air-glass interface as shown.  The plane of incidence contains the normal to the boundary and the incident ray.  The electric field vector E of the incident wave is perpendicular to the direction of propagation and can have a component in the plane of incidence, E_p, and a component perpendicular to the plane of incidence E_s.  We have E = E_p+ E_s.

The reflectance R depends of the polarization and is given for p-polarization by

R_p = ((tan(θ_i - θ_t)/tan(θ_i + θ_t))²,

and for s-polarization by

R_s = ((sin(θ_i - θ_t)/sin(θ_i + θ_t))².

If θ₁ + θ₂ = π/2, then tan(θ₁ + θ₂) = infinite and R_p = 0.  If light is reflected, it will have s-polarization.  The incident angle at which this happens is called the Brewster angle θ_B.  We then have

n₁sinθ_B = n₂sin((π/2) -θ_B) = n₂cosθ_B.
tanθ_B = n₂/n₁.

Explore using this spreadsheet.  Vary n1 and n2 and observe the changes.

Open a Microsoft Word document to keep a log of your procedures, results and discussions.  This log will become your lab report.  Address the points highlighted in blue.  Answer all questions.

Exploration

Use an on-line simulation from the University of Colorado PhET group to explore the bending of light.
Link to the simulation: https://phet.colorado.edu/en/simulations/bending-light

Explore the interface!

• Tools and objects can be dragged out of the tool box and then returned.
• The objects in the Prism tab can be rotated by dragging the handle.
• In the Prism Break tab, the protractor rotates and the laser translates.
• All the tools work in both Ray and Wave mode, but some are easier to use in Wave mode because the region where the tool can read is larger.

(a)  Click on either the Intro or More Tools tab.  Let red light move from air into water. (Make sure you select water if you click the More Tools tab.)
For incident angles θ_i from to zero 80^o in 10 degree steps measure the angle of refraction θ_t and the reflected intensity or reflectance R.
Download this spreadsheet and enter your measured values on sheet 1.

• Plot R versus theta (deg).  Paste your graph into your log.  Compare to the graph above.
• Discuss your result.
  • Is the laser light p-polarized, s-polarized, or unpolarized.
  • What do your results suggest?
• Calculate sinθ_i and sinθ_t.  Remember that Excel functions require the angles to be in radians.
  The first row if the spreadsheet already contains the formulas.
• Plot sinθ_i versus sinθ_t.
  • What does the plot look like?
  • Use the trendline to find the slope.  Paste the graph with trendline into your log.
  • What value do you obtain for the slope?
  • Given Snell's law, what value do you expect for the slope?  Discuss!

(b)  Design experiments to determine the index of refraction of mystery materials A and B.

• Describe your procedure and discuss why you decided to proceed this way.  What are your results for n_A and n_B?

(c)  Design and describe a setup that has the refracted ray bend away from the normal? 

• Paste a screen shot of your setup into your log.

(d)  Click on the prism tab.  Use red light with a wavelength of 650 nm.  Try to arrange various prisms in such a way, so that the laser beam after total internal reflections moves parallel to the incident beam but in the opposite direction.  Try to use as few pieces as possible.

• Paste a screen shot of your design into your log.

(e)  Now switch to white light and experiment with various prisms to answer the following questions.

• Are the reflection and refraction of light color-dependent?  How can you tell?  (Make sure you also show reflection by clicking the checkbox.)
• Which shapes split the white light into different colors the best?  Did you find a set-up that demonstrates this well?
• Try to arrange a situation so that the light light forms a rainbow.  What shape did you choose?

Experiment:

In this experiment you will trace the path of a light ray through a block of glass and measure the index of refraction of the glass.  For an air-glass boundary we can set the index of refraction of air equal to one.  Snell's law therefore yields for the index of refraction of the glass

n_glass = sinθ_air/sinθ_glass. 

It is easy to measure θ_air, but not θ_glass inside the glass.  But when a light ray enters square block of glass from air making a nonzero angle θ_air with the normal to the interface, the ray is bent towards the normal as its enters and away from the normal as it leaves the glass block.  The emergent ray moves in the same direction as the incident ray, but is displaced parallel to the incident ray.  The parallel displacement d depends on index of refraction n_glass and the width w of the block.  By measuring the displacement d, we can determine n_glass.

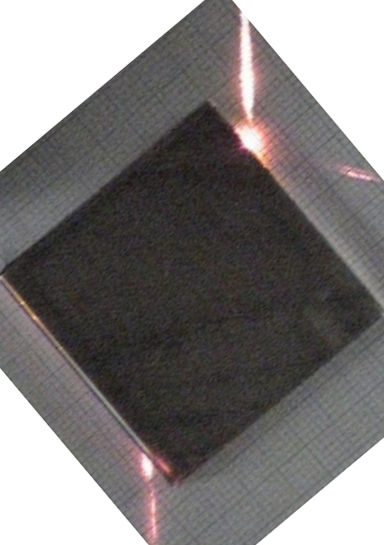
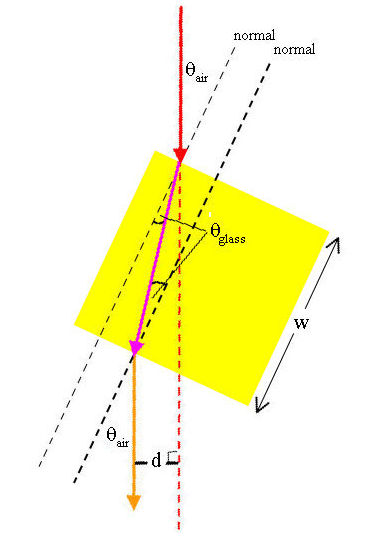
Procedure:

Click to open the "optics lab" simulation.  It contains a laser, an optical breadboard and several optical components.  The components can be dragged to different positions on the breadboard and the components can snap to the holes on the breadboard which have a spacing of 1 unit = 2.5 cm.  The screen is 4 units wide and 2 units high and the lines on the screen are spaced by ½ unit.  Clicking anywhere on the breadboard you can rotate and zoom the view.

When the simulation opens the laser beam passes through a glass block.  The dimensions of the glass block are 2.8 by 2.8 by 1.4 units.

• Drag the glass block out of the way and position the screen so that the undeflected beam hits the middle of the screen.
• Keep the rotation angle of the glass block at 0^o and drag the glass block back into position so that the laser beam passes through the block and hits the middle of the screen.
• Click the "Rotate postholder" button and rotate the glass block until the laser beam is displaced by d = 1/4 unit.  Remember that the lines on the screen are spaced by ½ unit.  Decrease the beam radius to its minimum value so that you can make a more precise measurement.  You can also drag the screen close to the glass block, and you can rotate an zoom the view.
• Record the angle θ_air corresponding to d = 1/4 unit in your spreadsheet on sheet 2.
• Repeat for d = ½ unit, 3/4 unit and 1 unit, for a total of 4 measurements.

Data Analysis:

Use your measurements to determine the index of refraction n_glass of the glass block.

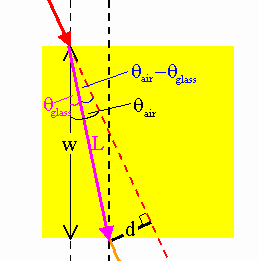The expected displacement of a ray passing through the glass block is d.  From the figure on the right we see that

d/L = sin(θ_air - θ_glass),
w/L = cos(θ_glass).

This can be rewritten as

d = wsin(θ_air - θ_glass)/cos(θ_glass),

and applying Snell's law and trigonometric relations

d = w sinθ_air[1 - cosθ_air/(n_glass² - sin²θ_air)^½].

This equation can be solved for n_glass.

 n_glass² = cos²θ_air/(1 - d/(w sinθ_air))² + sin²θ_air.

Use your spreadsheet to calculate n_glass for each of your 4 measurements.  Paste your spreadsheet table into your log.

• Do your measurements agree with each other within reasonable uncertainty, given how you performed your measurements.
• Do you suspect some of the measurements to be more accurate than others?  If yes, why?
• From your measurements, what do you conclude is the index of refraction n + Δn of the glass block?

Convert your log into a lab report.  See the grading scheme for all lab reports.

Name:
E-mail address:

Laboratory 10 Report

• In one or two sentences, state the goal of this lab.
• Make sure you completed the entire lab and answered all parts.  Make sure you show your work and inserted and properly labeled relevant tables and plots.
• Add a reflection at the end of your report in a short essay format.

Save your Word document (your name_lab10.docx), go to Canvas, Assignments, Lab 10, and submit your document.