There is another way to make sure two interfering waves have the same polarization.  We reflect the wave from the two surfaces of a thin film.  This is called division of amplitude.

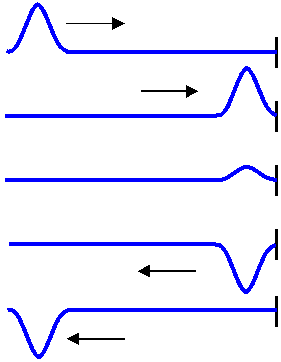When the medium through which a wave travels abruptly changes, the wave may be partially or totally reflected.  When studying mechanical waves we found that when a wave pulse traveling along a rope reaches the end of the rope, it is totally reflected.  The details of the reflection depend on if the end of the rope is tied down and fixed, or if it is allowed to swing loose.
A wave pulse, which is totally reflected from a rope with a fixed end is inverted upon reflection. The phase shift of the reflected wave with respect to the incident wave is π or 180^o.

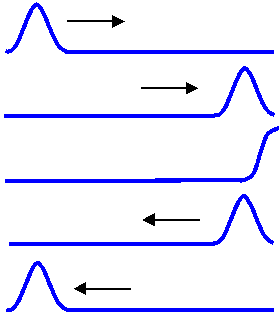A wave pulse, which is totally reflected from a rope with a loose end is not inverted upon reflection.  The phase shift of the reflected wave with respect to the incident wave is zero.  When a periodic wave is totally reflected, then the incident wave and the reflected wave travel in the same medium in opposite directions and interfere.

We observe a similar phenomenon with light waves.  When a light wave reflects from a medium with a larger index of refraction, then the phase shift of the reflected wave with respect to the incident wave is π or 180^o.  When a light wave reflects from a medium with a smaller index of refraction, then the phase shift of the reflected wave with respect to the incident wave is zero. 

Thin-film interference is the interference of light waves reflecting off the top surface of a thin film with the waves reflecting from the bottom surface.   Constructive and destructive interference of reflected light waves causes the colorful patterns we often observe in thin films, such as soap bubbles and layers of oil on water.  If the thickness of the film is on the order of the wavelength of light, then colorful patterns can be obtained, as shown in the image on the right.

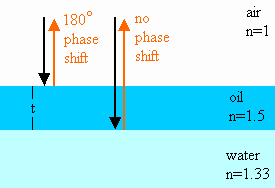Consider the case of a thin film of oil of thickness t floating on water.  For simplicity, assume that the light is incident normally, so that the angle of incidence and the angle of reflection are zero

In the air, the light reflecting off the air-oil interface will have a 180^o phase shift with respect to the incident light.  A 180^o phase shift is equivalent to the light having traveled a distance of ½ wavelength.  In the oil, the light reflecting from the oil-water interface will have no phase shift with respect to the light incident on the interface.  For the light reflected off the oil and the light reflected off the water to constructively interfere we need the two reflected waves to have a phase shift of an integer multiple of 2π or 360^o.  If the light reflected off the oil-water interface travels an additional distance equal to ½ the wavelength of the light in oil, then the total phase shift with respect to the light reflected off the air-oil interface will be 2π.  This happens if the thickness t of the film is equal to 1/4 the wavelength of the light in oil, since the light has to traverse this thickness twice.  We also get constructive interference if the thickness of the film is equal to 3/4, 5/4, ..., the wavelength of the light in oil.  For constructive interference we need

2t = (m+½)λ_n,  m = 0,1,2,...,

where λ_n is the wavelength of the light in oil.
In vacuum we have λf = c.  In a medium with index of refraction n we have λ_nf = c/n.  The frequency of oscillation is the same in vacuum and in a medium, therefore

λ_n= λ/n.

For constructive interference we therefore need

2 n_oil t = (m+½)λ, m = 0,1,2,....

Destructive interference occurs when the thickness of the oil film is equal to (½)λ_n, λ_n, (3/2)λ_n, etc.
For destructive interference we therefore need

2 n_oil t = mλ, m = 0,1,2,....

If the thickness of the film is (1/4)λ_n = (1/4)λ/n_oil the phase of the wave reflected off the top surface is shifted by π by the reflection.  The phase of the wave traveling through the film is not shifted by reflection off the bottom surface, but the wave travels an extra distance of λ_n/2.  It will therefore be in phase with the wave reflected off the top surface.  If, on the other hand, the film thickness is ½λ_n, =  ½λ/n_oil then the wave traveling through the film travels an extra distance of one wavelength.  It will therefore be out of phase with the wave reflected off the top surface and the two waves will cancel each other out.

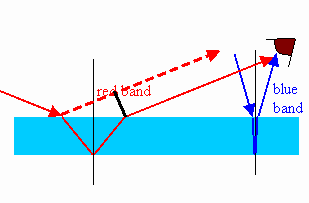Waves incident at an angle θ_i on the air oil interface are refracted as they enter the oil.  The angle of refraction θ_t is found from Snell's law, n_airsinθ_i = n_oilsinθ_t.  If they are reflected off the second interface, then they travel a distance 2t/cosθ_t in the oil.  When they emerge again from the oil into the air and propagate parallel to the waves reflected at the air-oil interface, then the total optical path length difference is

2 n_oil t/cosθ_t - 2t tanθ_tsinθ_i = 2 n_oil t/cosθ_t - 2t tanθ_t(n_oil/n_air)sinθ_t
= (2n_oilt/cosθ_t)(1 - sin²θ_t) = 2 n_oil t cosθ_t.

For constructive interference we therefore need

2 n_oil t cosθ_t = (m+½)λ, m = 0,1,2,...,

and for destructive interference we need

2 n_oil t cosθ_t = mλ, m = 0,1,2,....

Constructive and destructive interference occur at different angles for different wavelength.  The observer sees colored bands.

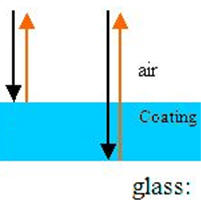
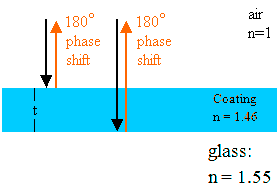Destructive interference of reflected light waves is utilized to make non-reflective coatings for light at near normal incidence.  Such coatings are commonly found on camera lenses and binocular lenses, and often have a bluish tint.  The coating is put over glass, and the coating material generally has an index of refraction less than that of glass.  Then the phase shift of both reflected waves is 180^o.  For such a coating on glass, at normal incidence, we need

2 n_coat t = mλ, m = 0,1,2,...,

and for destructive interference we need

2 n_coat t = (m+½)λ, m = 1,2,....

For a film thickness equal to t = (1/4)λ/n_coat we get a net shift of ½ wavelength between the wave reflected from the top and bottom of the film, resulting in cancellation.  For a non-reflective coatings the minimum film thickness required is (1/4)λ/n_coat.  A coating with thickness t = λ/4n_coat prevents the reflection of most of the light with a wavelength λ close to λ = 4n_coatt.  The coating does not reflect a specific range of wavelengths.  Often that range is chosen to be in the yellow-green region of the spectrum, where the eye is most sensitive.  Lenses coated for the yellow-green region reflect in the blue and red regions, giving the surface a familiar purple color.

Problem:

When sunlight reflects from a thin film of soapy water, the film appears multicolored, in part because destructive interference removes different wavelengths from the light reflected at different places, depending on the thickness of the film.  As the film becomes thinner and thinner, it looks darker and darker in reflected light, appearing black just before it breaks.  The blackness means that destructive interference removes all wavelengths from the reflected light when the film is very thin.  Explain.

Solution:

• Reasoning:
  The phase shift of a ray in air reflecting from the air-film interface is π.  The phase shift of a ray traveling through the film and reflecting from the film-air interface is zero.  As the film gets thinner, most of the phase difference between the two reflected rays is due to π radians phase shift during the reflection off the air-film interface.  This phase shift is the same for all wavelengths, and results in destructive interference.

Problem:

A thin film of a material is floating on water (n = 1.33).  When the material has a refractive index of n = 1.20, the film looks bright in reflected light as its thickness approaches zero.  But when the material has a refractive index of n = 1.45, the film looks black in reflected light as the thickness approaches zero.  Explain these observations in terms of constructive and destructive interference and the phase changes that occur when light waves undergo reflection.

Solution:

• Reasoning:
  If the refractive index of the film is greater than that of the water below, the phase shift of the ray traveling through the film and reflecting off the interface between the film and the water is zero.  We have the same situation as in the previous problem.
  If the refractive index of the film is less than that of water the phase shift of the ray traveling through the film and reflecting off the interface between the film and the water is π.  Both reflected rays now have the same phase.  As the film thickness approaches zero, they are in phase and the material will look bright.

The colorful patterns that you see when light reflects off a compact disk are produced by thin film interference.

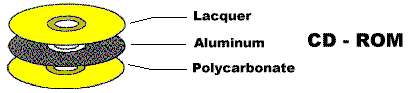
A compact disc is made of a polycarbonate wafer which is coated with a metallic film, usually an aluminum alloy.  The aluminum film is then covered by a plastic polycarbonate coating.  The coatings are less than 100 nm thick and each coating partially reflects and partially transmits incident light.  Light rays reflected from different coating boundaries interfere with each other to produce the colorful patterns.  The reflectance of the CD is not uniform, because CD disk contains a long string of pits written helically on the disk.  These pits encode the information stored on the CD.

Thin film interference is used in industry as a non-contact, non-destructive way to measure film thicknesses.

External links:  Thin film interference (part 1)     Thin film interference (part 2)  (Youtube)