Bernoulli's equation

P₁ + ρgh₁ + ½ρv₁² = P₂ + ρgh₂ + ½ρv₂²
or
P + ρgh + ½ρv² = constant.

A liquid at rest  (hydrostatics)

If a liquid is at rest, then

P₁ + ρgh₁  = P₂ + ρgh₂,
P_bottom + ρgh_bottom = P_top + ρgh_top,
P_bottom = P_top + ρg(h_top - h_bottom).

The pressure in the fluid increases linearly with depth.

If a liquid is at rest in a horizontal pipe, P₁  = P₂, the pressure is the same everywhere.

A liquid flowing in a horizontal pipe

P₁ + ½ρv₁² = P₂ + ½ρv₂².

If a liquid (or a gas which is not being compressed) is flowing frictionless in a steady state through a horizontal pipe with a varying cross-sectional area, then the pressure depends on the speed of the fluid.

The faster the fluid is flowing, the lower is the pressure at the same height.

This may seem counterintuitive to you, but it is a consequence of conservation of energy.  The molecules of a fluid at room temperature are always in motion, even if the fluid as a whole is at rest.  This disordered motion is responsible for the pressure exerted by the fluid, even in gravity-free space.  In a pipe, it results in collisions with the walls.  If a fluid is flowing trough a horizontal pipe at a steady rate, then the molecules also have ordered motion.  In a narrow section of the pipe the fluid is flowing faster, and more of its energy goes into the ordered motion.  This leaves less energy for the random motion and therefore results in softer collisions and lower pressure.  Phenomena which can be understood with the help of Bernoulli's equation include the Pitot tube, the Venturi effect, atomizers, hurricanes, flapping flags, etc.

Faster flow --> more kinetic energy in the ordered motion --> less kinetic energy in the random motion --> lower pressure

Problem:

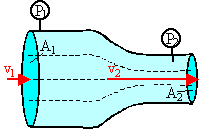A Venturi tube may be used as a fluid flow meter.  If the difference in pressure P₁ - P₂ = 21 kPa, find the fluid flow rate in m³/s given that the radius of the outlet tube is 1 cm, the radius of the inlet tube is 2 cm, and the fluid is gasoline (ρ = 700 kg/m³).
 

Solution:

• Reasoning:
  From Bernoulli's equation we have P₁ + ρgh₁ + ½ρv₁² = P₂ + ρgh₂ + ½ρv₂²,. 
  h is constant, so P₁ + ½ρv₁² = P₂ + ½ρv₂², or P₁ - P₂ = ½ρv₂² - ½ρv₁².
  From the equation of continuity we have
  Area 1*v₁ = Area 2*v₂.  v₁ = (A₂/A₁)v₂.
• Details of the calculation:
  Using P₁ - P₂ = ½ρv₂² - ½ρv₁², we have
  21 kPa = 350 kg/m³ (v₂² - v₁²).
  Inserting  v₁ = (A₂/A₁)v₂. into the above equation we have
  (1-(A₂/A₁)²)v₂²) = (21000/350)(m/s)².
  (A₂/A₁)² = (1/4)² = 1/16.  v₂² = (21000/350)(16/15)(m/s)² = 64(m/s)^2..
  v₂ = 8 m/s.
  Volume flow rate: A₂v₂ = π(0.01 m)²*8 m/s = 0.0025 m³/s.

Real fluids are not ideal fluids.  But in a short enough section, the laminar flow of a real fluid may be approximately treated as ideal, if the energy loss in this section is very small compared to the ordered kinetic energy of the fluid in the section.  Then Bernoulli's equation is approximately valid for this section of the real fluid.

Embedded Question 1

You roll down the window on your car while driving on the freeway.  An empty plastic bag on the floor promptly flies out the window.  Explain why.

Discuss this with your fellow students in the discussion forum!