Nuclear energy can be produced by either of two types of reactions, fission, the splitting apart of a massive atomic nucleus, and fusion of lighter nuclei into a heavier nucleus. E = mc2. Whenever a system looses energy, it looses mass. Let us compare the energy released per kg of fuel for various energy-releasing reactions.
| Energy Source |
Chemical | Fission | Fusion |
|---|---|---|---|
| Sample Reaction |
C + O2 --> CO2 |
n + 235U --> 143Ba + 91Kr + 2n |
2H + 3H --> 4He + n |
| Typical Inputs (to Power Plant) |
Bituminous Coal |
UO2 (3% 235U + 97% 238U) |
Deuterium & Lithium |
| Typical Reaction Temperature (K) |
700 |
1000 |
108 |
| Energy Released per gram of Fuel (J/kg) |
3.3 x 107 |
2.1 x 1012 |
3.4 x 1014 |
| Efficiency (E/mc2) |
3 * 10-8% |
0.002% |
0.4% |

 A stream of water can broken up into a
series of droplets by vibrating the hose out of which the stream
emerges. Using a strobe light, we can stop the apparent motion of
the individual droplets. The result is a strobe photograph of the
motion of the droplets. We can follow the motion of an individual
drop. Adjusting the strobe frequency so that the drop appears to
fall slowly, we can watch an individual drop oscillate as it falls.
Oscillations of one drop are shown in the figure on the left. The
droplet model of Bohr and Wheeler suggests that similar oscillations
take place in a large nucleus like Uranium, particularly if the nucleus
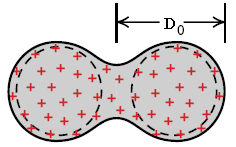
is struck by a particle, such as a slow neutron. Suppose we have an oscillating Uranium
nucleus, and at a particular time it has the dumbbell shape shown.
A stream of water can broken up into a
series of droplets by vibrating the hose out of which the stream
emerges. Using a strobe light, we can stop the apparent motion of
the individual droplets. The result is a strobe photograph of the
motion of the droplets. We can follow the motion of an individual
drop. Adjusting the strobe frequency so that the drop appears to
fall slowly, we can watch an individual drop oscillate as it falls.
Oscillations of one drop are shown in the figure on the left. The
droplet model of Bohr and Wheeler suggests that similar oscillations
take place in a large nucleus like Uranium, particularly if the nucleus
is struck by a particle, such as a slow neutron. Suppose we have an oscillating Uranium
nucleus, and at a particular time it has the dumbbell shape shown.
In this shape we have two spheres, outlined by dotted circles, connected by a neck of nuclear matter. The distance between the spheres is greater than D0, the range of the nuclear force. The electrostatic repulsion between the spheres is stronger than the nuclear attraction. The only thing that holds this nucleus together is the neck of nuclear matter between the spheres.
If the Uranium nucleus is struck too vigorously and the neck is stretched too far, the electrostatic force will cause the two ends to fly apart, releasing a huge quantity of electrostatic potential energy.
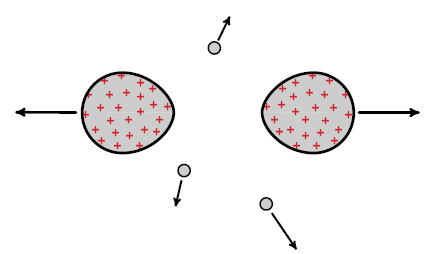
The process is called nuclear
fission. In the fission of Uranium 235, the large Uranium nucleus
breaks up into two moderate sized nuclei, for example, Cesium 140 and
Zirconium 94. Because larger nuclei have a higher percentage of
neutrons than smaller ones, the break-up of Uranium into smaller, less
neutron rich nuclei results in the emission of free neutrons.
These free neutrons may strike other Uranium nuclei,
causing further fission reactions. If in small block of Uranium
235 one of the Uranium nuclei fissions spontaneously, the extra free
neutrons are likely to leave through the edges of the block without
inducing further fissions. If, however, the block is large enough,
if it exceeds a critical mass of
about 52 kg for a sphere (diameter ~17 cm), then neutrons from one
nucleus that fissions spontaneously are more likely to strike other
Uranium nuclei than to escape. The result is that several other
nuclei fission, and each of these cause several others to fission.
Quickly you have a large number of nuclei which fission in a process
called a chain reaction. This is the process that occurs in an
uncontrolled way in an atomic bomb and in a controlled way in a nuclear
reactor. The energy we get from nuclear fission, the energy from
all commercial nuclear reactors, is electrostatic potential energy which
is converted into kinetic energy when the two nuclear fragments fly
apart. The fragments which are only connected by a neck of nuclear
matter are well beyond the range D0 of the attractive nuclear
force, and essentially feel only the repulsive electric force between
the protons. These two balls of positive charge have a large positive
electric potential energy which is converted to kinetic energy as the
fragments fly apart.
To get a feeling for the amount of energy released in a fission reaction,
calculate the electrostatic potential energy of two fragments, say a Cesium and
a Zirconium nucleus when separated by a distance 2D0, twice the range
of the nuclear force. (D0 is
approximately 4 times the proton diameter, D0 = 4*1.4*10-15
m.)
Solution:
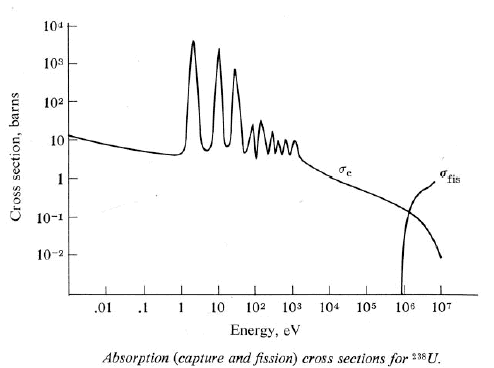
Reactors based on the fission of 235U by thermal neutrons use
enriched uranium. Natural uranium contains 0.7% of the isotope 235U,
the remaining 99.3% being 238U, which is not
fissionable by thermal
neutrons. The reactor fuel is artificially enriched so that it contains ~3%
235U. In a reactor the operators must be able to control the likelihood
that a released neutron produces another fission. A working reactor has to be
designed to overcome 3 problems.
(a) The Neutron Leakage Problem
Some of the neutrons produced by fission will leak out of the reactor and not
contribute to the chain reaction. Leakage is a surface effect. Neutron
production occurs throughout the volume of the fuel. The fraction of neutrons
lost by leakage can be reduced by using a large reactor core, thereby reducing
the surface to volume ratio of the fuel.
(b) The Neutron Energy Problem
The neutrons produced by fission are fast, with kinetic energies of about 2 MeV. To effectively induce fission, thermal neutrons are needed. The fast neutrons
can be slowed down by mixing the uranium fuel with a moderator which slows down
neutrons via elastic collisions and does not absorb neutrons and remove them
from the core. Most power reactors in North America use water as a moderator. The hydrogen nuclei in the water have approximately the same mass as the fast
neutrons. In a head-on elastic collisions with a proton, a neutron can loose
nearly all its kinetic energy.
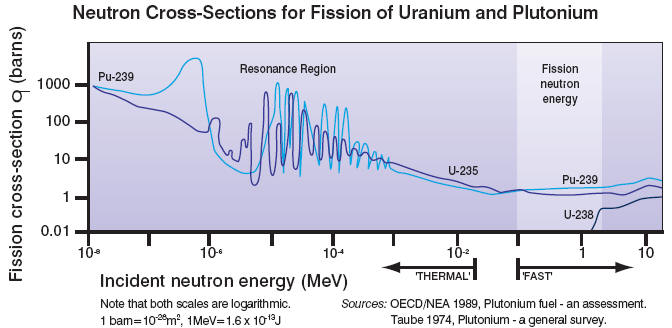
(c) The Neutron Capture Problem
As the fast neutrons (~2 MeV) are slowed down in the moderator to thermal
energies (~0.04 eV), they must pass through a critical energy interval (~1 to
100 eV) in which they are likely be capture by 238U nuclei. Such
non-fission resonance capture removes the neutron from the fission chain. To
minimize non-fission capture, the uranium fuel and the moderator are placed into
different regions of the reactor volume. Still, approximately 1/4 of the
neutrons are lost to non-fission capture.
In a typical reactor, the uranium fuel is in the form of uranium oxide pellets, which are inserted into long, hollow metal tubes. The liquid moderator surrounds bundles of these fuel rods, forming the reactor core. This arrangement increases the probability that a fast neutron, produced in a fuel rod, will find itself in the moderator when it passes through the critical energy interval.
 |
 |
 ; ; |
| Fuel pellet | Fuel-rod bundle | Reactor core |
When a reactor is operating at a steady power level, exactly the same number
of excess neutrons is produced in a given time interval by fission in the reactor core
as is lost by leakage or non-fission capture in the same time interval.
The multiplication factor k is the ratio of the number of neutrons
present after a fission event that go on to cause another fission event. When
the reactor is operating at a steady power level k = 1, it is said to be
critical. Reactors are designed so that they are inherently supercritical (k >
1) and the multiplication factor is then adjusted to k = 1 by inserting control
rods into the reactor core. These rods are made from materials such as cadmium
that absorb neutrons readily. The rods can be inserted farther to reduce the
operating power level and withdrawn to increase the power level.
A small percentage (~0.65%) of the neutrons are released by the fission
fragments with a delay of up to 55 s (14 s average). The reactor is only
critical when these neutrons also have a chance of inducing new fissions. This
allows time for the control rods to be adjusted to control the power.
Find the disintegration energy Q for the fission event n + 235U
--> 140Xe + 94Sr + 2n taking into account the decay of the
fission fragments. 40Xe and 94Sr are both highly unstable
and undergo beta decay until a stable end product is reached. In each beta decay
a neutron decays into a proton and an electron and antineutrino are emitted.
For 140Xe the decay chain is
140Xe --> 140Cs --> 140Ba --> 140La
--> 140Ce ,
and for 94Sr it is
94Sr --> 94Y --> 94Zr.
The atomic and particle masses are:
235U: 235.0439 u, 140Ce: 139.9054 u, 94ZZr: 93.9063 u, n: 1.00866 u.
Solution:
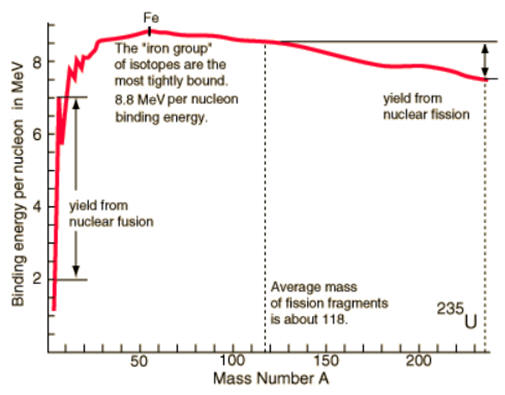
The process of combining small nuclei to form larger ones is called nuclear fusion. From a graph of nuclear binding energies we see that nuclear fusion releases energy if the resulting nucleus is smaller than Iron 56, but costs energy if the resulting nucleus is larger. This fact is significant in the life of stars and the formation of the elements. Most stars form from a gas cloud rich in hydrogen gas. When the cloud condenses, gravitational potential energy is released and the gas heats up. If the condensing cloud is massive enough and the temperature becomes hot enough, the hydrogen nuclei begin to fuse. After several reactions they produce Helium 4 nuclei, releasing energy in each reaction. The fusion of hydrogen to form helium becomes the source of energy for the star for many years to come. Unlike fission, fusion requires high temperatures in order to take place. Consider the reaction in which two hydrogen nuclei fuse to produce a deuterium nucleus plus a positron and a neutrino. When the two protons fuse, the resulting nucleus immediately gets rid of its electrical potential energy by β+ decay. One proton decays into a neutron, a positron, and a neutrino. The fusion of the two protons will take place if the protons get closer together than the range of the nuclear force, about four proton diameter. Before they get that close they repel each other. Only if the protons are initially moving fast enough can they get close enough to overcome the electrostatic repulsion. A graph of the potential energy of the approaching proton as a function of separation r is shown below.
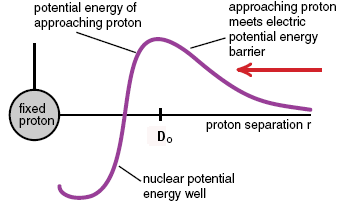
When the proton separation r is greater than the range D0 of the nuclear force, the protons repel and the incoming proton has to climb a potential energy hill. At R = D0, the net force turns attractive and the potential energy begins to decrease, forming a deep well. Energy is released when the incoming proton falls into the well, but the incoming proton must have enough kinetic energy to get over the potential energy barrier before fusion can take place.
Make a rough estimate of the kinetic energy and the temperature required for fusion of two protons to take place.
Solution:
Two billion Kelvin is a huge overestimate. At this temperature, the average proton in the gas would enter into a fusion reaction. If we heated a container of hydrogen to this temperature, the entire collection of protons would fuse after only a few collisions, and the fusion energy would be released almost instantaneously. We would have a hydrogen bomb. In a star, the fusion of hydrogen takes place at the much lower temperatures of about 20 million degrees. At 20 million degrees, only a small fraction of the protons have enough kinetic energy to enter into a fusion reaction. Some of these protons with energy just below the energy needed to overcome the barrier tunnel through the barrier. At 20 million degrees the hydrogen is consumed at a slow steady rate in what is known as a controlled fusion reaction.
The Sun emits approximately 4*1026 Watts of energy. For comparison, the US Power consumption is approximately 3*1013 Watts. The energy output of the sun is approximately equivalent to what would be produced by exploding > 100 billion nuclear bombs per second. We know from dating of rocks on the Earth and Moon that the Sun is at least 4.5 billion years old, so it has emitted about 6*1043 J of energy during its lifetime. Chemical reactions or gravitational contraction cannot account for this energy.
The Proton-Proton Chain is the principal set of reactions for solar-type stars to transform hydrogen to helium:
1H + 1H --> 2H + e+ + neutrino
Two protons react to form deuterium plus a positron and a neutrino. In the highly ionized stellar interior the positron will quickly "annihilate" with an electron (e+ + e- --> 2 gamma-rays). The gamma-rays will be absorbed and re-emitted by the dense matter in the stellar interior, gradually diffusing outward and being "degraded" into photons of lower energy. When the gamma-ray energy reaches the photosphere each gamma-ray will have been transformed into about 200,000 visible photons. The neutrino, which only interacts through the weak force, streams straight out of the sun.
2H + 1H --> 3He + gamma-ray
The deuterium reacts with another proton to form 3He plus another gamma-ray. The first two reactions must happen twice to form two 3He nuclei.
3He + 3He --> 4He + 2 1H
The two 3He nuclei react to form 4He and two
protons and kinetic of the product nuclei. Note that the 3He
nuclei repel each other more strongly because they contain two positively
charged protons. The initial reactions in the chain can occur at
temperatures as low as 1 million K, but the last reaction can only occur at
temperatures greater than about 10 million K.
The individual nuclear reactions proceed rather slowly. A
very small fraction of nuclei in the core of the sun has enough kinetic energy
to overcome the electrostatic repulsion. Even so, every second the sun
turns 600 million tons of hydrogen into 596 million tons of helium, with 4
million tons transformed into luminous energy via E = mc2.
Overall the p-p cycle combines 4 protons
and two electrons to form an
alpha particle, two neutrinos, and
6 gamma rays. The energy released in the
overall reaction is Q = 26.7 MeV. Approximately 0.5 MeV is carried away by
neutrinos, 26.2 MeV is eventually carried away by electromagnetic radiation.
The p-p Chain
| Reactions |
Illustration |
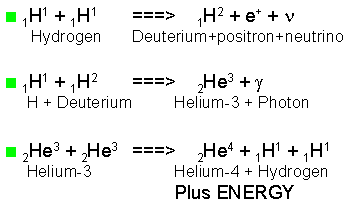 |
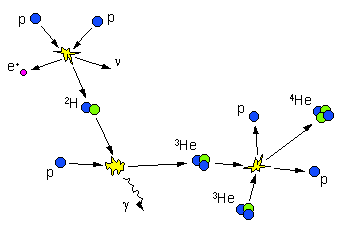 |